Read custom data in UE4

Posted on February 14, 2021February 14, 2021 by Giorgos Kourakos

I have recently started looking into ways to use Unreal Engine 4 for my visualizations and so far it looks quite promising. The data I usually work come from groundwater modeling simulations, therefore are not typical data one gets from modeling software such as Blender Houdini etc.

So the following is my way to get data from custom ascii files to UE4. For this guide I will assume that the data are organized as follows:

Nnodes Ntriangles
X Y Z1 Z2 Z3
.
.
.
ID1 ID2 ID3
.
.
.

Nnodes Ntriangles

X Y Z1 Z2 Z3

ID1 ID2 ID3

The first line is the number of nodes and the number of triangles. The procedure I’m following accepts only triangular data. so I have actually converted the quadrilaterals to triangles when I created the above file. Then Nnodes times is repeated the line that holds the x y coordinates. Note that in the file I provide 3 z coordinates. These correspond to ground surface, water table and bottom of the aquifer. in my case. Then I’m listing the ids of each of the Ntriangles of the mesh.

Inside UE4 I started from a C++ project and created an class named CustomMesh based on Actor class. The header file is the following

Header file

#pragma once

#include "CoreMinimal.h"
#include "GameFramework/Actor.h"
#include "ProceduralMeshComponent.h"
#include "CustomMesh.generated.h"

UCLASS()
class BUILING_ESCAPE_API ACustomMesh : public AActor
{
	GENERATED_BODY()
	
public:	
	// Sets default values for this actor's properties
	ACustomMesh();

protected:
	// Called when the game starts or when spawned
	virtual void BeginPlay() override;

	virtual void OnConstruction(const FTransform& Transform) override;

public:	
	// Called every frame
	virtual void Tick(float DeltaTime) override;
	UPROPERTY(VisibleAnywhere, BlueprintReadWrite)
	UProceduralMeshComponent* PM;

	UPROPERTY(EditAnywhere, Category= "DATA")
	FString InputFile;
	
	UPROPERTY(EditAnywhere, Category= "DATA")
	float XYScale = 1000.f;

	UPROPERTY(EditAnywhere, Category= "DATA")
	float ZScale = 10.f;
};

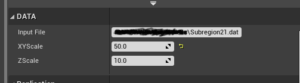
#pragma once

#include "CoreMinimal.h"

#include "GameFramework/Actor.h"

#include "ProceduralMeshComponent.h"

#include "CustomMesh.generated.h"

UCLASS()

class BUILING_ESCAPE_API ACustomMesh : public AActor

{

GENERATED_BODY()

public:

// Sets default values for this actor's properties

ACustomMesh();

protected:

// Called when the game starts or when spawned

virtual void BeginPlay() override;

virtual void OnConstruction(const FTransform& Transform) override;

public:

// Called every frame

virtual void Tick(float DeltaTime) override;

UPROPERTY(VisibleAnywhere, BlueprintReadWrite)

UProceduralMeshComponent* PM;

UPROPERTY(EditAnywhere, Category= "DATA")

FString InputFile;

UPROPERTY(EditAnywhere, Category= "DATA")

float XYScale = 1000.f;

UPROPERTY(EditAnywhere, Category= "DATA")

float ZScale = 10.f;

};

The most important line in the header file is the OnConstruction virtual method which is called every time the asset is transformed. Apart from the virtual method I defined 3 exposed properties InputFile, XYscale, Zscale. There is also a UProceduralMeshComponent that holds the actual data. This actually should not be exposed however I did it as I’m still experimenting with this. This header file will create a category DATA as shown below where the data can be updated inside the editor.

Implementation file
- Constructor

#include "CustomMesh.h"
#include "Misc/FileHelper.h"

// Sets default values
ACustomMesh::ACustomMesh()
{
 	// Set this actor to call Tick() every frame.  You can turn this off to improve performance if you don't need it.
	PrimaryActorTick.bCanEverTick = true;
	
	PM = CreateDefaultSubobject<UProceduralMeshComponent>(TEXT("Procedural mesh"));
	SetRootComponent(PM);
	PM->bUseAsyncCooking = true;

}

#include "CustomMesh.h"

#include "Misc/FileHelper.h"

// Sets default values

ACustomMesh::ACustomMesh()

{

// Set this actor to call Tick() every frame. You can turn this off to improve performance if you don't need it.

PrimaryActorTick.bCanEverTick = true;

PM = CreateDefaultSubobject<UProceduralMeshComponent>(TEXT("Procedural mesh"));

SetRootComponent(PM);

PM->bUseAsyncCooking = true;

}

In the constructor all I’m doing is to create the object and set it as root. Note also that I have included the Filehepler.h which is used for reading the custom file.

- OnConstruction

First we clear out and existing mesh in the object and initialize required variables.

PM->ClearAllMeshSections();
TArray<FString> FileContent;
FVector LowerCorner(999999999.f,999999999.f,999999999.f);
FVector UpperCorner(-999999999.f,-999999999.f,-999999999.f);
FVector Center(0.f, 0.f,0.f);
int Nverts = 0;
int Ntris = 0;
int iline = 0;

FVector T = Transform.GetLocation();

TArray<FVector> GSEVertices;
TArray<FVector> BOTVertices;
TArray<FVector> WTVertices;
TArray<FVector> Normals;
TArray<int32> Indices;
TArray<FColor> ColorVert;

PM->ClearAllMeshSections();

TArray<FString> FileContent;

FVector LowerCorner(999999999.f,999999999.f,999999999.f);

FVector UpperCorner(-999999999.f,-999999999.f,-999999999.f);

FVector Center(0.f, 0.f,0.f);

int Nverts = 0;

int Ntris = 0;

int iline = 0;

FVector T = Transform.GetLocation();

TArray<FVector> GSEVertices;

TArray<FVector> BOTVertices;

TArray<FVector> WTVertices;

TArray<FVector> Normals;

TArray<int32> Indices;

TArray<FColor> ColorVert;

Then I read the first line to get the number of vertices and number of triangles.

const bool bTF = FFileHelper::LoadANSITextFileToStrings(*InputFile,NULL, FileContent);
if (bTF){
    if (FileContent.Num() > 0){
	//Read the number of vertices and triangles
	TArray<FString> Out;
	FileContent[iline].ParseIntoArray(Out, TEXT(" "), true);
	iline++;
	if (Out.Num() > 1){
	    Nverts = FCString::Atoi(*Out[0]);
	    Ntris = FCString::Atoi(*Out[1]);
	}
     }

const bool bTF = FFileHelper::LoadANSITextFileToStrings(*InputFile,NULL, FileContent);

if (bTF){

if (FileContent.Num() > 0){

//Read the number of vertices and triangles

TArray<FString> Out;

FileContent[iline].ParseIntoArray(Out, TEXT(" "), true);

iline++;

if (Out.Num() > 1){

Nverts = FCString::Atoi(*Out[0]);

Ntris = FCString::Atoi(*Out[1]);

}

Next we read the vertices. Note that while reading the x y coordinates we identify the bounding box of the data so that we can translate the mesh into the center of the transform. Otherwise the mesh will be located somewhere far away of the gizmo icon.

if (FileContent.Num() > Nverts)
{
    for (int i = 0; i < Nverts; ++i)
    {
	TArray<FString> Out;
	FileContent[iline].ParseIntoArray(Out, TEXT(" "), true);
	iline++;
	if (Out.Num() > 4)
	{
   	    float x = FCString::Atof(*Out[0])/XYScale + T.X;
	    float y = FCString::Atof(*Out[1])/XYScale + T.Y;
	    float t = FCString::Atof(*Out[2])/ZScale + T.Z;
	    float w = FCString::Atof(*Out[3])/ZScale + T.Z;
	    float b = FCString::Atof(*Out[4])/ZScale + T.Z;
	    if (x > UpperCorner.X) UpperCorner.X = x;
	    if (y > UpperCorner.Y) UpperCorner.Y = y;
	    if (t > UpperCorner.Z) UpperCorner.Z = t;
	    if (x < LowerCorner.X) LowerCorner.X = x;
	    if (y < LowerCorner.Y) LowerCorner.Y = y;
	    if (t < LowerCorner.Z) LowerCorner.Z = t;
	    GSEVertices.Add(FVector(x,y,t));
	    Normals.Add(FVector::UpVector);
	    BOTVertices.Add(FVector(x,y,b));
	    WTVertices.Add(FVector(x,y,w));
	    ColorVert.Add(FColor::MakeRandomColor());
	}
    }
    Center = (UpperCorner + LowerCorner)/2;
    for (int i = 0; i < GSEVertices.Num(); ++i)
    {
	GSEVertices[i] = GSEVertices[i] - Center;
	BOTVertices[i] = BOTVertices[i] - Center;
	WTVertices[i] = WTVertices[i] - Center;
    }
}

if (FileContent.Num() > Nverts)

{

for (int i = 0; i < Nverts; ++i)

{

TArray<FString> Out;

FileContent[iline].ParseIntoArray(Out, TEXT(" "), true);

iline++;

if (Out.Num() > 4)

{

float x = FCString::Atof(*Out[0])/XYScale + T.X;

float y = FCString::Atof(*Out[1])/XYScale + T.Y;

float t = FCString::Atof(*Out[2])/ZScale + T.Z;

float w = FCString::Atof(*Out[3])/ZScale + T.Z;

float b = FCString::Atof(*Out[4])/ZScale + T.Z;

if (x > UpperCorner.X) UpperCorner.X = x;

if (y > UpperCorner.Y) UpperCorner.Y = y;

if (t > UpperCorner.Z) UpperCorner.Z = t;

if (x < LowerCorner.X) LowerCorner.X = x;

if (y < LowerCorner.Y) LowerCorner.Y = y;

if (t < LowerCorner.Z) LowerCorner.Z = t;

GSEVertices.Add(FVector(x,y,t));

Normals.Add(FVector::UpVector);

BOTVertices.Add(FVector(x,y,b));

WTVertices.Add(FVector(x,y,w));

ColorVert.Add(FColor::MakeRandomColor());

}

Center = (UpperCorner + LowerCorner)/2;

for (int i = 0; i < GSEVertices.Num(); ++i)

{

GSEVertices[i] = GSEVertices[i] - Center;

BOTVertices[i] = BOTVertices[i] - Center;

WTVertices[i] = WTVertices[i] - Center;

}

Next we read the triangles. The triangles are stored as 1D vector.

if (FileContent.Num() > Nverts + Ntris)
{
    for (int i = 0; i < Ntris; ++i)
    {
	//UE_LOG(LogTemp, Warning, TEXT("%d"), i);
	TArray<FString> Out;
	FileContent[iline].ParseIntoArray(Out, TEXT(" "), true);
	iline++;
	if (Out.Num() > 2)
	{
	    Indices.Add(FCString::Atoi(*Out[0])-1);
	    Indices.Add(FCString::Atoi(*Out[2])-1);
	    Indices.Add(FCString::Atoi(*Out[1])-1);
	}
    }
}

if (FileContent.Num() > Nverts + Ntris)

{

for (int i = 0; i < Ntris; ++i)

{

//UE_LOG(LogTemp, Warning, TEXT("%d"), i);

TArray<FString> Out;

FileContent[iline].ParseIntoArray(Out, TEXT(" "), true);

iline++;

if (Out.Num() > 2)

{

Indices.Add(FCString::Atoi(*Out[0])-1);

Indices.Add(FCString::Atoi(*Out[2])-1);

Indices.Add(FCString::Atoi(*Out[1])-1);

}

Last we create the custom meshes. For each Z coordinate I create one mesh, which set to visible after the creation.

PM->CreateMeshSection_LinearColor(0, GSEVertices, Indices, TArray<FVector>(), TArray<FVector2D>(), TArray<FLinearColor>(), TArray<FProcMeshTangent>(),true);
PM->CreateMeshSection_LinearColor(1, WTVertices, Indices, TArray<FVector>(), TArray<FVector2D>(), TArray<FLinearColor>(), TArray<FProcMeshTangent>(),true);
PM->CreateMeshSection_LinearColor(2, BOTVertices, Indices, TArray<FVector>(), TArray<FVector2D>(), TArray<FLinearColor>(), TArray<FProcMeshTangent>(),true);
	
/*PM->CreateMeshSection(0, Vertices, Indices,
	Normals,
	TArray<FVector2D>(),
	ColorVert,
	TArray<FProcMeshTangent>(),
	true);*/
PM->SetMeshSectionVisible(0,true);
PM->SetMeshSectionVisible(1,true);
PM->SetMeshSectionVisible(2,true);

PM->CreateMeshSection_LinearColor(0, GSEVertices, Indices, TArray<FVector>(), TArray<FVector2D>(), TArray<FLinearColor>(), TArray<FProcMeshTangent>(),true);

PM->CreateMeshSection_LinearColor(1, WTVertices, Indices, TArray<FVector>(), TArray<FVector2D>(), TArray<FLinearColor>(), TArray<FProcMeshTangent>(),true);

PM->CreateMeshSection_LinearColor(2, BOTVertices, Indices, TArray<FVector>(), TArray<FVector2D>(), TArray<FLinearColor>(), TArray<FProcMeshTangent>(),true);

/*PM->CreateMeshSection(0, Vertices, Indices,

Normals,

TArray<FVector2D>(),

ColorVert,

TArray<FProcMeshTangent>(),

true);*/

PM->SetMeshSectionVisible(0,true);

PM->SetMeshSectionVisible(1,true);

PM->SetMeshSectionVisible(2,true);

So far this is adequate to create the custom mesh. However created a blueprint class based on the Custom Mesh class which I dragged into my scene.

The above code does not read the UV coordinates. Soon I’ll add this info in the file and show some figures.

To be continued…

Subplots in Matlab

Posted on January 20, 2021 by Giorgos Kourakos

One very annoying thing in Matlab subplots is the large space around each subplot.
So the following is a way to overcome this.

To be fair, the credits for the solution goes to … (have a look at this link)

In short the following code, which has been copied from the link above, produces very nice and tight subplots

clf
nRows = 2 ;
nCols = 2 ;
figure() ;
set( gcf, 'Units', 'normalized', 'Position', [0.1, 0.1, 0.5, 0.8] ) ;
[blx, bly] = meshgrid( 0.05:0.9/nCols:0.9, 0.05:0.9/nRows:0.9 ) ;
hAxes = arrayfun( @(x,y) axes( 'Position', [x, y, 0.9*0.9/nCols, 0.9*0.9/nRows] ), blx, bly, 'UniformOutput', false ) ;
for k = 1 : numel( hAxes )
    axes( hAxes{k} ) ;
    surf(peaks(40));
    set( gca, 'Visible', 'on' ) ;
end

clf

nRows = 2 ;

nCols = 2 ;

figure() ;

set( gcf, 'Units', 'normalized', 'Position', [0.1, 0.1, 0.5, 0.8] ) ;

[blx, bly] = meshgrid( 0.05:0.9/nCols:0.9, 0.05:0.9/nRows:0.9 ) ;

hAxes = arrayfun( @(x,y) axes( 'Position', [x, y, 0.9*0.9/nCols, 0.9*0.9/nRows] ), blx, bly, 'UniformOutput', false ) ;

for k = 1 : numel( hAxes )

axes( hAxes{k} ) ;

surf(peaks(40));

set( gca, 'Visible', 'on' ) ;

end

Reading IWFM/C2VSim Output files

Posted on December 9, 2020January 19, 2021 by Giorgos Kourakos

The latest version of IWFM uses hdf output file format. This makes the reading output files a bit more difficult since the outputs are not in ASCII format but way more efficient. Luckily Matlab, R and many other languages can handle hdf formats quite easily.

Groundwater Zone Budget file

The first step to read any hdf file in matlab is to read the information that is contained in the file

GB_bud_info = h5info(fullfile(c2vsim_path, 'Results','C2VSimFG_GW_ZBudget.hdf'));

1	GB_bud_info = h5info(fullfile(c2vsim_path, 'Results','C2VSimFG_GW_ZBudget.hdf'));

The GB_bud_info is a structure with many fields that contain plenty of useful information. After examining the structure in my version the GB_bud_info contained the field Groups, which has the following structure

If we expand the dataset for the Layer1 we see the following structure

From this table we can find the row where each property is located . To read the data for a specific property we use the following. The Storage for example is the row 22. The following snippet loops through the layers and adds the entire storage from all layers

CVstorage = zeros(504, 32537);
for ii = 1:4
    CVstorage = CVstorage + h5read(GB_bud_info.Filename,...
    [GB_bud_info.Groups(1+ii).Name GB_bud_info.Name GB_bud_info.Groups(1+ii).Datasets(22).Name])';
end

CVstorage = zeros(504, 32537);

for ii = 1:4

CVstorage = CVstorage + h5read(GB_bud_info.Filename,...

[GB_bud_info.Groups(1+ii).Name GB_bud_info.Name GB_bud_info.Groups(1+ii).Datasets(22).Name])';

end

The units of the hdf file are in feet. Since this is storage the units are cubic feet. To generate the famous storage depletion plot of Central valley for example after we have calculated the above we can do the following

% Create the time variable for the plot
start_date = datetime(1973,10,1);
tm = start_date + calmonths(0:503);
% Calculate the storage from all elements
CVcumulativeStorage = sum(CVstorage,2);
% convert from feet to acre feet
CVcumulativeStorage = CVcumulativeStorage * 2.29569e-5
% Convert to million acrefeet
CVcumulativeStorage = CVcumulativeStorage /1000000
% and do the plot
plot(tm,CVcumulativeStorage - CVcumulativeStorage(1),'linewidth',2) 
xlabel('Time')
ylabel('Storage depletion [MAF]')
grid on

% Create the time variable for the plot

start_date = datetime(1973,10,1);

tm = start_date + calmonths(0:503);

% Calculate the storage from all elements

CVcumulativeStorage = sum(CVstorage,2);

% convert from feet to acre feet

CVcumulativeStorage = CVcumulativeStorage * 2.29569e-5

% Convert to million acrefeet

CVcumulativeStorage = CVcumulativeStorage /1000000

% and do the plot

plot(tm,CVcumulativeStorage - CVcumulativeStorage(1),'linewidth',2)

xlabel('Time')

ylabel('Storage depletion [MAF]')

grid on

In the case of storage the data have dimensions as we see in the ChunkSize field 32537 and we can somewhat safely assume that the 1st row correspond to 1st element etc. However the ChunkSize for the Streams (rows 23,24) is 9885.

StreamBud=h5read(GB_bud_info.Filename,...
    [GB_bud_info.Groups(2).Name GB_bud_info.Name GB_bud_info.Groups(2).Datasets(23).Name])';

1 2	StreamBud=h5read(GB_bud_info.Filename,... [GB_bud_info.Groups(2).Name GB_bud_info.Name GB_bud_info.Groups(2).Datasets(23).Name])';

The size of the StreamBud variable is 504×9885. Therefore from this matrix we cannot tell which element corresponds to the values. This information exists under Group(1).Datasets

The 6th row contains the correspondence between data and elements. To read it use

ids = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(6).Name])';

1	ids = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(6).Name])';

which returns a matrix 24×32537 (note that the above code transpose the output). Obviously the columns are related to the elements but how about the rows? This information exists in the 5th row of the above variable which we can read it as

Colnames = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(5).Name]);

1	Colnames = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(5).Name]);

The Colnames variable consist of 24 rows! The 3rd row is the Streams_Inflow (+). The elements that are associated with the stream budget are the nonzero ones in the 3rd row of the ids matrix which can be extracted easily

stream_elem_ids = find(ids(3,:) ~=0);

1	stream_elem_ids = find(ids(3,:) ~=0);

Extract Budget terms for a sub-region

In the following we are going to extract the groundwater pumping for the 21st subregion. Note that this is not a continuation of the above

First we read the mesh information where the correspondence between subregions and mesh element is defined. For the following I’m using the latest version of the modeling which can be cloned from this repo. I also use one custom matlab function to read the element file which can be found in here

c2vsim_path = fullfile('..','C2VsimV1','c2vsim-working');
GB_bud_info = h5info(fullfile(c2vsim_path, 'Results','C2VSimFG_GW_ZBudget.hdf'));
c2vsim_mesh = readIWFM_Elements(fullfile(c2vsim_path,'Preprocessor',"C2VSimFG_Elements.dat"), 32537, 142);

c2vsim_path = fullfile('..','C2VsimV1','c2vsim-working');

GB_bud_info = h5info(fullfile(c2vsim_path, 'Results','C2VSimFG_GW_ZBudget.hdf'));

c2vsim_mesh = readIWFM_Elements(fullfile(c2vsim_path,'Preprocessor',"C2VSimFG_Elements.dat"), 32537, 142);

The pumping is not printed for all elements and not all layers pump from the same elements. The following is looping through the 4 layers of the models and extract which elements are associated with the budget terms. We also read the column names

Colnames = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(5).Name]);
for ii = 1:4
    ids{ii,1} = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(5+ii).Name])';
end

Colnames = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(5).Name]);

for ii = 1:4

ids{ii,1} = h5read(GB_bud_info.Filename,[GB_bud_info.Groups(1).Name GB_bud_info.Name GB_bud_info.Groups(1).Datasets(5+ii).Name])';

end

In this version the column names consists of 26 rows and the rows 21-24 are related to pumping. However we are interested only in the negative terms which denote extraction from the groundwater. Besides the positive pumping is zero in the model. The rows 22 and 24 correspond to element and well pumping.

Before assembling the budget terms we can find the elements of the subregion of interest. Here I’ll use the 21st subregion

isub = 21;
subreg_elem = find(c2vsim_mesh(:,end) == isub);

1 2	isub = 21; subreg_elem = find(c2vsim_mesh(:,end) == isub);

The next snippet loops through the layers and extracts the element ids for the element and well pumping. If any element has no pumping then the id will be 0 so we remove the 0 entries. Last we read the data from the hdf5 file and sum the pumping from all the elements for each time step and save the results to a matrix.

elem_pump = zeros(504, 4);
well_pump = zeros(504, 4);
for ii = 1:4
    elem_pump_ind = ids{ii,1}(22,subreg_elem);
    well_pump_ind = ids{ii,1}(24,subreg_elem);
    elem_pump_ind(:,elem_pump_ind==0) = [];
    well_pump_ind(:,well_pump_ind==0) = [];
    tmp_elem_pmp = h5read(GB_bud_info.Filename, [GB_bud_info.Groups(1+ii).Name GB_bud_info.Name GB_bud_info.Groups(1+ii).Datasets(13).Name])';
    elem_pump(:,ii) = sum(tmp_elem_pmp(:,elem_pump_ind),2);
    tmp_well_pmp = h5read(GB_bud_info.Filename, [GB_bud_info.Groups(1+ii).Name GB_bud_info.Name GB_bud_info.Groups(1+ii).Datasets(15).Name])';
    well_pump(:,ii) = sum(tmp_well_pmp(:,well_pump_ind),2);
end

elem_pump = zeros(504, 4);

well_pump = zeros(504, 4);

for ii = 1:4

elem_pump_ind = ids{ii,1}(22,subreg_elem);

well_pump_ind = ids{ii,1}(24,subreg_elem);

elem_pump_ind(:,elem_pump_ind==0) = [];

well_pump_ind(:,well_pump_ind==0) = [];

tmp_elem_pmp = h5read(GB_bud_info.Filename, [GB_bud_info.Groups(1+ii).Name GB_bud_info.Name GB_bud_info.Groups(1+ii).Datasets(13).Name])';

elem_pump(:,ii) = sum(tmp_elem_pmp(:,elem_pump_ind),2);

tmp_well_pmp = h5read(GB_bud_info.Filename, [GB_bud_info.Groups(1+ii).Name GB_bud_info.Name GB_bud_info.Groups(1+ii).Datasets(15).Name])';

well_pump(:,ii) = sum(tmp_well_pmp(:,well_pump_ind),2);

end

Now let’ s plot the pumping for each layer separately

start_date = datetime(1973,10,1); 
tm = start_date + calmonths(0:503); 
plot(tm, elem_pump * 2.29569e-5/1000)
xlabel('Time')
ylabel('Pumping [TAF]')
legend('Layer 1','Layer 2','Layer 3','Layer 4')

start_date = datetime(1973,10,1);

tm = start_date + calmonths(0:503);

plot(tm, elem_pump * 2.29569e-5/1000)

xlabel('Time')

ylabel('Pumping [TAF]')

legend('Layer 1','Layer 2','Layer 3','Layer 4')

Subsurface

George Kourakos

Uncategorized

Read custom data in UE4

Subplots in Matlab

Reading IWFM/C2VSim Output files

Groundwater Zone Budget file

Extract Budget terms for a sub-region